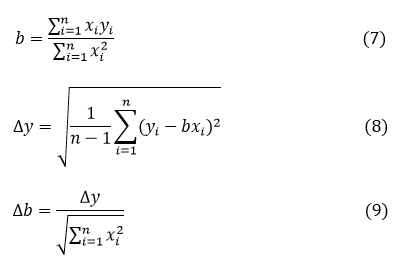PRACTICAL WORK OF PHYSICS (MECHANICS)
2. REMINDER ON THE LINEAR REGRESSION METHOD
We use the linear regression method only when the absolute uncertainty on \( x \) is \( ( \Delta x \approx 0 ) \) negligible and the absolute uncertainty on is constant \( ( \Delta y = cste ) \) .
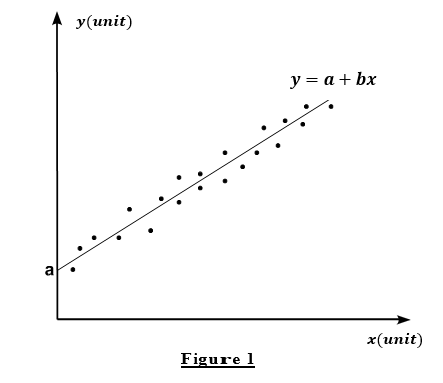
This method consists in drawing the best possible straight line of equation \( y=a+bx \) which passes as close as possible to all the experimental points, as illustrated in figure 1.
\( b \) represents the slope of the regression line while is the ordinate at the origin.
Knowing that we have \( n \) measurements performed in practical work, then the values of \( a \) and \( b \) are given by the formulas:
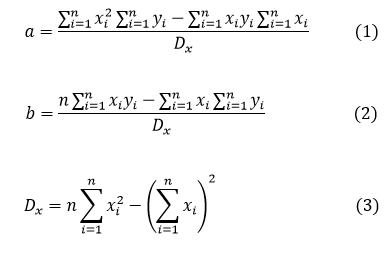
The uncertainties associated with each parameter are given by:
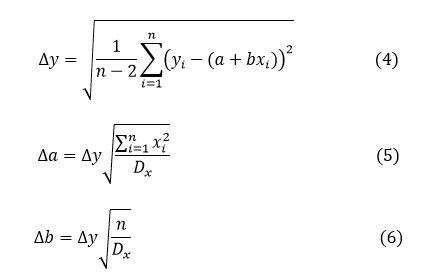
If the linear regression line passes through the origin, we have the equation of the line \( y=bx \), in this case \(a=0\) and we obtain: